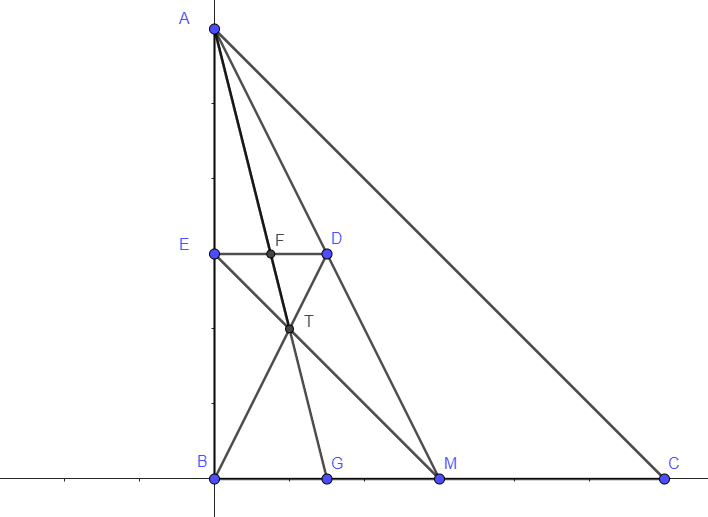
Let TG be a median of triangle BMT
And triangle BMT is similar to triangle DET
So FT is a median of triangle DET
Then the area of traingle FDT = (1/2) [ DET ] = 7
And triangle AED similar to triangle ABM
So ED = 1/2 BM
And FD = 1/2 GM
So the height of triangle FDT = 1/2 height of triangle GTM
So FT / FG = FT / [ FT + 2FT] = 1/3
So FT = (1/3)FG
FG = 3FT
And FG = AF
So AF = 3 FT
So the height of triangle AFD = 3 times the height of triangle FDT
And since they're on the same base (ED)..... the area of triangle AFD = 3 [ FDT] = 3 * 7 = 21
[ ADT ] = [ AFD ] + [ FDT ] = 21 + 7 = 28


