CPhill

(n + 1)^2 = n^2 + 2n + 1

            n

n + 2  [ n^2 + 2n + 1 ] 

            n^2 + 2n

            ___________

                             1

Note that this will never produce an integer because we will always have a remainder of  1 / (n + 2)

5*6*7*8 + 1  =  1681

630 / 42  = 15

Either   3 and 5    or     1 and  15

x^2 + 12x - 4 = 0

Let the roots be a,b

ab  = -4

2ab = -8

a + b = -12       square both sides

a^2 + 2ab + b^2 =144

a^2 - 8 + b^2  = 144

a^2 + b^2 = 152

x + y = 3 + k

y = -x + (3 + k)

The slope of the line = -1

The slope of a tangent line at any point on the circle  = -x/y

Set the slopes equal

-x/y = -1

-x= -y

x = y

So....subbing into both equations

x + x = 3 + k  →  2x = 3 + k  →  x = (3 + k)/2   (1)

x^2 + x^2 = k  →  2x^2 = k   (2)

Sub (1)  into (2)

2 [ (3 + k)/2]^2 = k           simplify

k^2 + 6k + 9  = 2k

k^2 + 4k + 9 = 0

k cannot be a real number because the discriminant =  -20

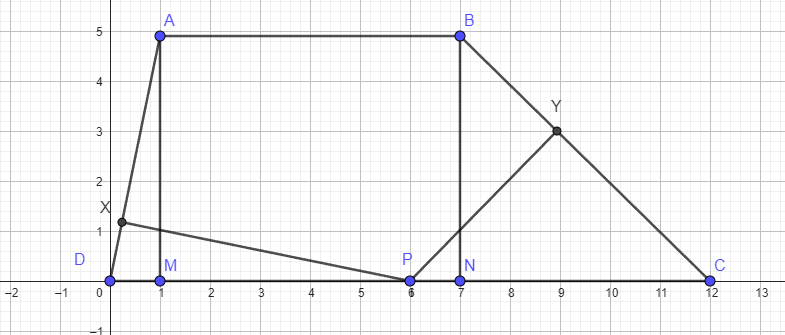
Draw AM , BN perp to CD

Let DN = x

CN = 12 - ( AB + DN)  =  12 - (6 + x)  =  6-x

AM = BN

So....By the Pythagorean Theorem

AD^2 - DM^2  = BC^2 - CN^2

5^2 - x^2  = 7^2 - ( 6-x)^2

25 - x^2 = 49 - x^2 + 12x - 36

25 =  12x + 13

12 = 12x

x =1 = DM

Note that we have two right triangles ADM  and PDX

Angles AMD and PXD are equal = 90°

And angle ADM = angle PDX

So  triangle ADM is similar to triangle PDX

PD / AD = 6/5

So

DX / DM = 6/5

DX = (6/5)DM = (6/5) (1) = 6/5

So

sqrt [DP^2 - DX^2] = PX  

sqrt [ 6^2 - (6/5)^2 ] =  sqrt [ 36*25 - 36 ] / 5 = sqrt [ 900 - 36] / 5  = sqrt [ 864] / 5  =

sqrt [ 144 * 6 ] / 5  =  12sqrt (6) / 5 = PX

Similarly

Right Triangles BCN and PCY are similar

PC/BC  = 6/7

So

CY / CN  = 6/7

CY / 5 = 6/7

CY = 30/7

So

sqrt [ PC^2 - CY^2 ] = PY

sqrt [ 6^2 - (30/7)^2] = sqrt [ 36 * 49 - 900 ] / 7  =  sqrt [ 1764 - 900] / 7 = sqrt [ 864]/7  =

sqrt [ 144 *6 ] / 7 = 12sqrt(6) / 7 = PY

Seems OK, NTS  !!!!

Here's a similar prob that I answered earlier :

https://web2.0calc.com/questions/geometry_2526

See if you can solve it now....

Note, NTS, that PQR can't be a triangle because

PQ + PR  = QR

But the triangle inequality says that

PQ + PR  must be > QR

Let P  have the coordinates  ( x , -x + 6)

PA^2  = ( x - 10)^2 + (- x + 6 - -12)^2  =  (x -10)^2 + ( -x + 18)^2

PO^2 = ( x - 2)^2 + ( -x + 6 - 8)^2 = (x -2^2 + ( -x - 2)^2

We want   PA^2 = PO^2....so....

(x -10)^2 + ( -x + 18)^2 = ( x -2)^2 + (-x -2)^2

x^2 -20x + 100 + x^2 - 36x + 324  =  x^2 -4x + 4 + x^2 +4x + 4

-56x + 424  =  8

-56x = -416

x = 416/56 = 52/7

y = - 52/7 + 6 =  -10/7 

P = (52/7, -10/7)